下面为大家整理的是4道SAT数学练习题的内容,后面都附有详细的答案解析。SAT数学考试是非常注重对考生实际运用知识点能力的考察的,所以大家在备考的时候,一定要多加练习才行。下面我们来看看详细内容吧。
Read the following SAT test question and then click on a button to select your answer.
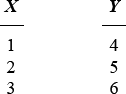
1.If x is a number from Column x and y is a number from Column y in the table above, how many different values are possible for x+y?
(A) Nine
(B) Eight
(C) Seven
(D) Six
(E) Five
Read the following SAT test question and then click on a button to select your answer.
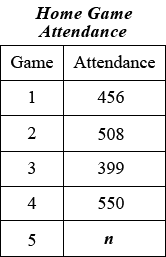
2.The table above shows the attendance at the home games of the Central High School football team. If the median attendance for the five games was 456, and no two games had the same attendance, what is the greatest possible value for n?
(A) 400
(B) 455
(C) 457
(D) 478
(E) 549
Read the following SAT test question and then click on a button to select your answer.
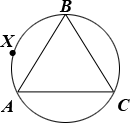
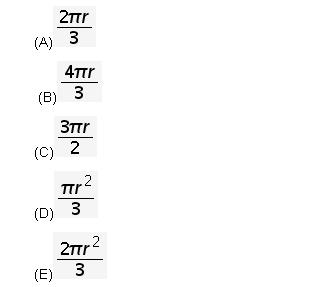
3.In the figure above, inscribed triangle ABC is equilateral. If the radius of the circle is r, then the length of arc AXB is
Read the following SAT test question and then click on a button to select your answer.
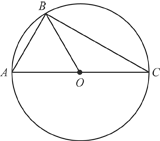
4.In the figure above, O is the center of the circle and triangle A B O is equilateral. If the sides of triangle A B O are of length 6, what is the length of line B C?
(A) 3 times square root 3
(B) 4 times square root 3
(C) 6 times square root 3
(D) 9
(E) 12

4.The correct answer is C
Choice (C) is correct. Since triangle A B O is equilateral, each of its angles has measure
60 degrees. It follows that angle B O C has measure 120 degrees. Since line O B and line O C are radii of the same circle, they are of equal length, and so delta O C B is isosceles. Hence angle O B C and angle O C B each have measure 30 degrees. Thus angle A B C has measure 60 degrees + 30 degrees = 90 degrees, and so triangle A B C is a 30 degrees minus 60 degrees minus 90 degrees right triangle. Since line A B, the side opposite the 30 degrees angle in triangle A B C, is of length 6, it follows that line B C, the side opposite the 60 degrees angle, is of length 6 times square root 3
以上就是这8道SAT数学练习题及答案的详细内容,包括了一些常见的知识点。大家可以在备考的时候,对此加以适当的练习和应用,测试自己在数学方面知识点的掌握情况,以便及时调整自己的备考方案,提高备考的效率。











